Explore the fundamentals of paraxial rays in geometric optics, their significance in precision modeling, and their role in modern optical design.
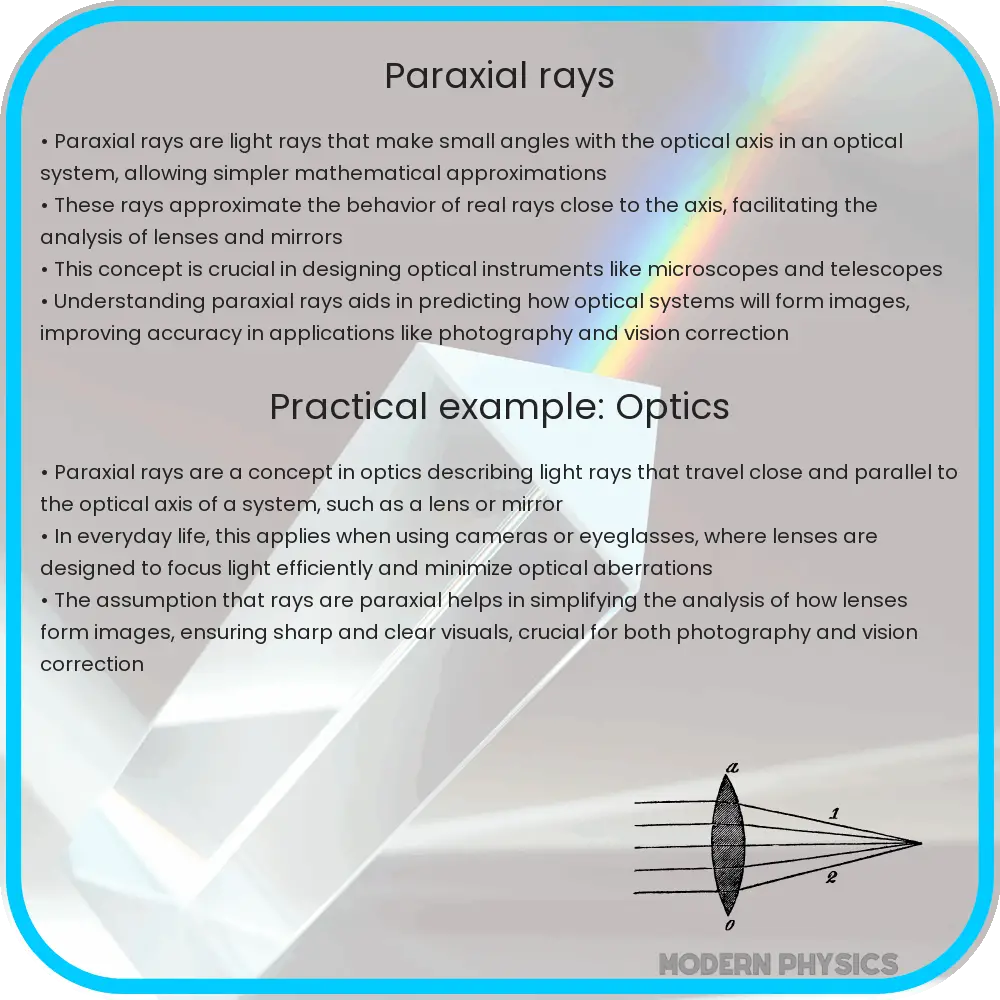
Understanding Paraxial Rays in Geometric Optics
Paraxial rays are a fundamental concept in the study of geometric optics, crucial for precision modeling and understanding light propagation. These rays, by definition, make small angles with the optical axis and are approximated to simplify the mathematical analysis of optical systems. This approximation allows for the derivation of simple, yet powerful, formulas to describe the behavior of optical systems such as lenses and mirrors.
The Significance of Paraxial Approximation
The paraxial approximation is instrumental in optical design and analysis, providing a streamlined approach to evaluating the performance of optical elements. By assuming that light rays are close to the axis and their angles of incidence and reflection are small, complex three-dimensional problems are reduced to more manageable two-dimensional ones. This simplification is key to designing lenses and mirrors with high precision, minimizing aberrations and enhancing image quality.
Modeling with Paraxial Rays
In the realm of geometric optics, modeling with paraxial rays involves using ray tracing techniques to predict how light interacts with optical surfaces. Ray tracing under the paraxial approximation involves linear equations, significantly simplifying the computational requirements. This method is particularly useful in the early stages of optical design, where the focus is on fundamental parameters like focal length, magnification, and image placement.
Applications in Optical Design
Paraxial rays play a crucial role in the design and analysis of optical systems, including cameras, telescopes, and microscopes. By employing the paraxial approximation, optical engineers can efficiently develop systems that achieve desired imaging properties. This approach is also vital in the correction of optical aberrations, where understanding the path of paraxial rays helps in designing elements that counteract deviations from ideal behavior.
Moreover, the study of paraxial rays extends beyond traditional optics, impacting fields such as laser physics and fiber optics. In these areas, the principles of paraxial approximation facilitate the design of systems for precise light transmission and manipulation, essential for telecommunications and medical technologies.
Challenges and Precision in Paraxial Ray Modeling
Despite its advantages, the paraxial approximation has its limitations, especially when dealing with high-precision optical systems. As the angle of incidence increases, or as the system demands greater accuracy, the simplifications of paraxial optics may lead to significant errors. Advanced optical design often requires moving beyond paraxial approximation to account for higher-order aberrations and non-linear effects. This necessitates more complex mathematical models and numerical methods, such as wave optics and ray tracing beyond the paraxial limit.
Advancements in Computational Optics
The field of computational optics has made significant strides in overcoming the limitations of paraxial ray modeling. Modern computational methods enable the simulation of optical systems with high accuracy, incorporating both paraxial and non-paraxial rays. These advancements allow for the precise design of optical components and systems, accommodating a wide range of applications from astronomical telescopes to microscopic imaging systems.
Integrating Paraxial Rays into Modern Optical Design
Despite the evolution of optical modeling techniques, the concept of paraxial rays remains a cornerstone in optical design. It provides a solid foundation for understanding the basic principles of light propagation and the initial stages of system design. As optical technologies advance, the integration of paraxial ray modeling with sophisticated computational techniques continues to be essential for developing innovative optical solutions.
Conclusion
Paraxial rays and the approximation they represent play a pivotal role in the field of geometric optics, simplifying the complex nature of light propagation. This foundational concept enables the efficient design and analysis of optical systems, from the simplest lenses to the most advanced telescopes. While the paraxial approximation has its limitations, the ongoing advancements in computational optics and the integration of more comprehensive modeling approaches ensure its continued relevance. As optical technology progresses, the principles underlying paraxial ray modeling remain indispensable for both understanding the fundamentals of optics and pushing the boundaries of optical design and application.
Is this conversation helpful so far?
