Scalar curvature is a key metric in general relativity that quantifies the curvature of space-time, derived from the Ricci curvature tensor and metric tensor.
Scalar Curvature: A Key Concept in General Relativity
In the realm of general relativity, developed by Albert Einstein, the concept of curvature plays a pivotal role in understanding the nature of space-time and gravity. Specifically, scalar curvature is a critical quantity that helps describe the curvature of space-time at a particular point. This article delves into what scalar curvature is and why it is essential in the context of general relativity.
What is Scalar Curvature?
Scalar curvature, often denoted by the symbol \(R\), is a measure of the curvature of space-time. It essentially represents the degree to which the geometry described by a metric deviates from that of flat space. In simple terms, it provides a single number that summarizes the curvature at a point in a way that is independent of the direction you look.
In the context of a two-dimensional surface, you can think of scalar curvature as analogous to the Gaussian curvature. For higher-dimensional spaces, this concept generalizes to provide information about the curvature of the space-time continuum.
Mathematical Representation
To understand scalar curvature from a mathematical perspective, one must consider the Riemann curvature tensor, denoted as \(R^{\rho}_{\sigma\mu\nu}\). The scalar curvature is derived from this tensor by performing specific contractions:
- First, we obtain the Ricci curvature tensor \(R_{\mu\nu}\) by contracting the Riemann tensor: \(R_{\mu\nu} = R^{\rho}_{\mu\rho\nu}\).
- Then, we contract the Ricci tensor to get the scalar curvature: \(R = g^{\mu\nu} R_{\mu\nu}\), where \(g^{\mu\nu}\) is the inverse of the metric tensor \(g_{\mu\nu}\).
Thus, the equation for scalar curvature can be written as:
\[
R = g^{\mu\nu} R_{\mu\nu}
\]
Significance in General Relativity
In Einstein’s field equations, scalar curvature is an integral part of the expression that links the geometry of space-time to the distribution of matter and energy. The field equations are given by:
\[
G_{\mu\nu} + \Lambda g_{\mu\nu} = \frac{8\pi G}{c^4} T_{\mu\nu}
\]
Here, \(G_{\mu\nu}\) is the Einstein tensor, \(\Lambda\) is the cosmological constant, \(G\) is the gravitational constant, \(c\) is the speed of light, and \(T_{\mu\nu}\) is the stress-energy tensor. The Einstein tensor, \(G_{\mu\nu}\), incorporates the Ricci curvature tensor and the scalar curvature:
\[
G_{\mu\nu} = R_{\mu\nu} – \frac{1}{2} R g_{\mu\nu}
\]
This portrayal emphasizes how scalar curvature, along with other forms of curvature, describes how matter and energy curve space-time.
Applications and Implications
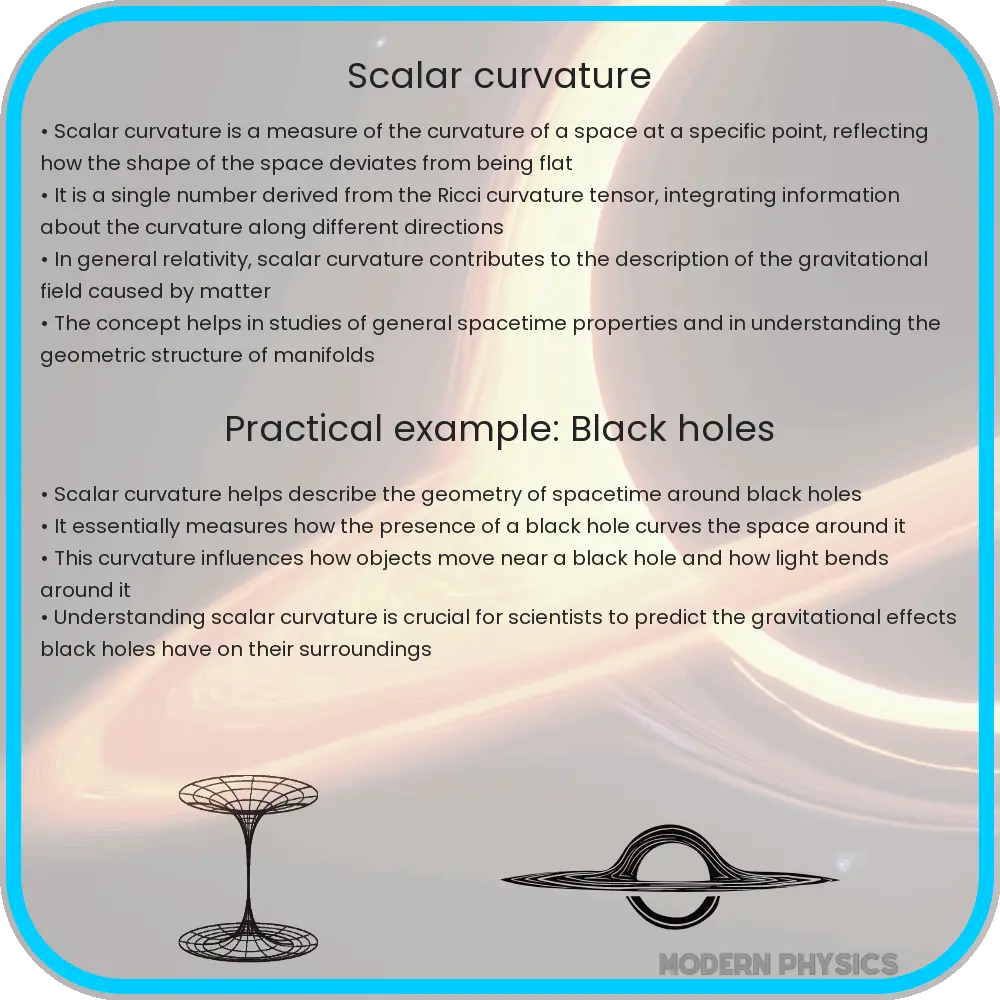
Scalar curvature appears in various solutions of Einstein’s field equations, such as the Schwarzschild solution for black holes and the Friedmann–Lemaître–Robertson–Walker (FLRW) metric for cosmology. These solutions help us understand extreme conditions in the universe, from the formation of black holes to the expansion of the universe.
Moreover, the sign of the scalar curvature reveals distinct geometric properties:
- Positive scalar curvature indicates space-time that curves like a sphere.
- Zero scalar curvature represents flat space-time.
- Negative scalar curvature signifies a saddle-shaped, hyperbolic geometry.
Visualization of Scalar Curvature
One effective way to grasp the concept of scalar curvature is through visualization. In a two-dimensional space, imagine a rubber sheet with various shapes:
- A perfectly flat rubber sheet represents zero scalar curvature.
- A rubber sheet bent into a dome shape exhibits positive scalar curvature.
- A rubber sheet shaped like a saddle shows negative scalar curvature.
In higher dimensions, these visualizations become abstract but the principles remain consistent: different shapes illustrate how space-time curves at every point.
Experimental Evidence
The theoretical predictions involving scalar curvature have been confirmed through various experiments and observations. For instance, the perihelion precession of Mercury’s orbit, the bending of light around massive objects, and the cosmological redshift observed in distant galaxies all serve as evidence that scalar curvature affects space-time in observable ways.
Conclusion
In summary, scalar curvature is a fundamental concept in general relativity, offering a quantitative measure of space-time curvature at any given point. Its significance is evident in Einstein’s field equations, which connect the geometry of our universe to the distribution of matter and energy within it. By exploring scalar curvature, we gain deeper insights into the structure of the cosmos, from the behavior of black holes to the dynamics of the universe’s expansion.
Understanding scalar curvature not only enhances our grasp of general relativity but also propels us further in comprehending the very fabric of our universe. As we continue to probe deeper into the mysteries of space-time, scalar curvature will undoubtedly remain an essential tool in the physicist’s toolkit.
