Explore the critical angle in optics: Understand refraction, total internal reflection, and their applications in technology and nature.
Critical Angle in Optics
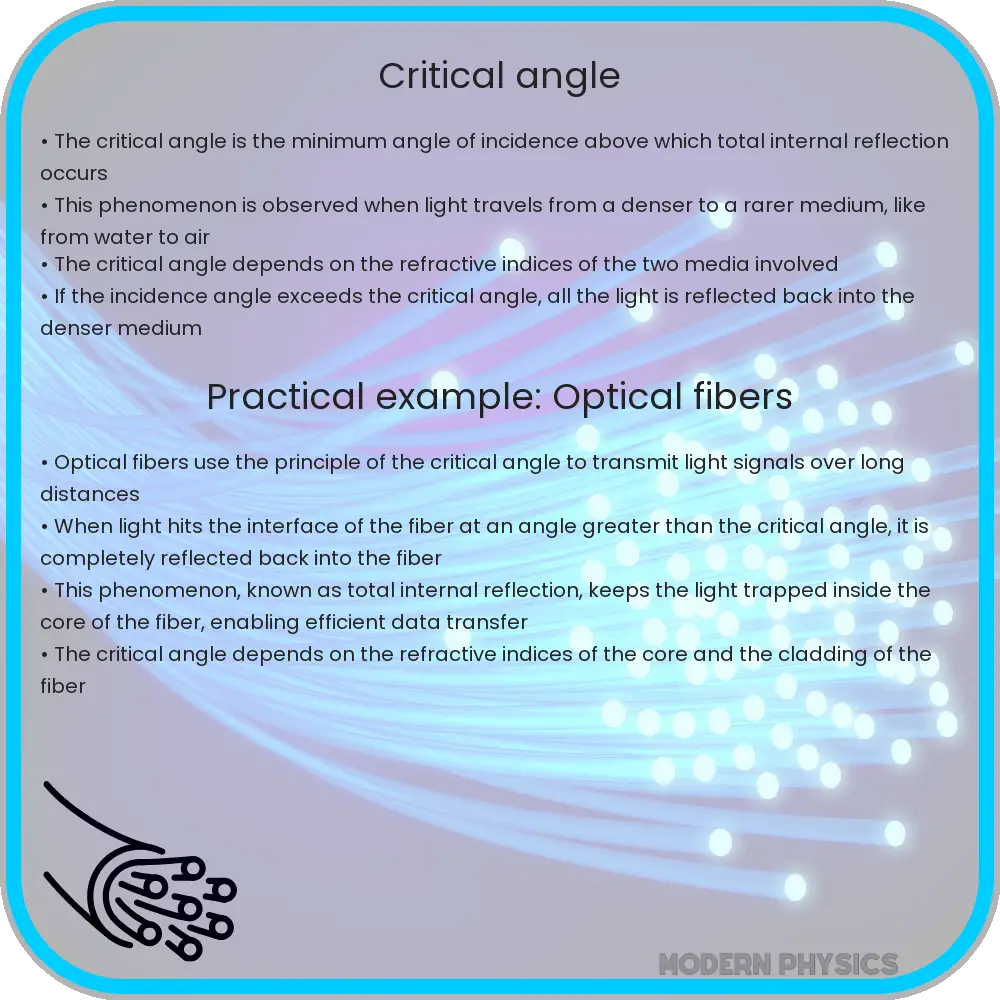
The concept of the critical angle is fundamental in the field of optics, particularly when discussing the phenomena of refraction and total internal reflection. It is crucial in understanding how light behaves when transitioning between different mediums.
Understanding Refraction
Refraction occurs when light passes from one medium into another, causing it to change direction. This bending of light is governed by Snell’s Law, which states:
n1sin(θ1) = n2sin(θ2)
Here, n1 and n2 are the refractive indices of the first and second mediums, respectively, and θ1 and θ2 are the angles of incidence and refraction.
Total Internal Reflection and the Critical Angle
Total Internal Reflection (TIR) is a phenomenon that occurs when light attempts to move from a denser medium to a less dense one at an angle greater than a certain threshold known as the critical angle. Beyond this angle, all the light is reflected back into the denser medium, rather than being refracted out of it.
The critical angle can be calculated using the formula:
θc = sin-1(n2/n1)
Where θc is the critical angle, and n1 and n2 are the refractive indices of the denser and less dense mediums, respectively. It’s important to note that this formula is only applicable when n1 is greater than n2.
Applications in Optics
The principles of the critical angle and TIR are widely applied in various optical technologies. For instance, fiber optic cables utilize TIR to efficiently transmit light over long distances with minimal loss. Similarly, prisms in binoculars and periscopes use these principles to reflect light within a confined space, enabling image magnification and direction change.
The study of critical angles enhances our understanding of natural phenomena like the shimmering effect at the bottom of a swimming pool and the formation of mirages in deserts. This knowledge is not only pivotal in physics but also in diverse fields such as telecommunications, astronomy, and even art.
Is this conversation helpful so far?
Factors Influencing the Critical Angle
The critical angle is not a fixed value and varies depending on several factors. The most significant factor is the refractive index of the mediums involved. The greater the difference in the refractive indices of the two mediums, the smaller the critical angle becomes. This variance explains why the critical angle for light moving from water to air is different from that of light moving from glass to air.
Impact of Wavelength on Critical Angle
Another interesting aspect is the influence of light’s wavelength on the critical angle. Different wavelengths of light refract differently, a phenomenon known as dispersion. This means that the critical angle can slightly vary depending on the color of the light, being slightly different for red light compared to blue light, for instance.
Practical Considerations
In practical applications, understanding and calculating the critical angle is essential. For instance, in designing optical instruments like microscopes and telescopes, engineers must consider the critical angles of the materials used to ensure optimal light transmission and minimal loss. In the field of photography, lens makers use these principles to design lenses that minimize aberrations caused by refraction.
Education and Research
The concept of critical angle also plays a vital role in education, particularly in physics and optics courses. It helps students understand the nature of light and its interaction with different materials. In research, scientists explore the critical angle to develop new materials with unique refractive properties, potentially leading to innovations in optical technology.
Conclusion
The concept of the critical angle, intertwined with the phenomena of refraction and total internal reflection, is a cornerstone of optics. It explains many natural and technological occurrences, from the basic principles of rainbows and mirages to the sophisticated design of fiber optics and lenses. The critical angle’s dependency on factors like the refractive index and wavelength of light underscores the intricate nature of light’s interaction with matter. Understanding and harnessing this knowledge continues to drive advancements in optical technologies, improving various aspects of our daily lives from communication to entertainment. The ongoing exploration of these principles promises further exciting developments in the field of optics and beyond.
